Today I'm going to show a set of renders that I did some time ago. The general idea is to make a spatial maze.
That is, one that allows movements upwards and downwards as well as forward, backward, right and left. The movements that a player can do are in any spatial axis. In this case, Theseus has to know how to climb well.
One simple is this one:
That is, one that allows movements upwards and downwards as well as forward, backward, right and left. The movements that a player can do are in any spatial axis. In this case, Theseus has to know how to climb well.
One simple is this one:

In this one we see a tridimensional labyrinth bounded by the surface of a cube.
The procedure to accomplish this renders is not complex.
Other views of the cube:
The procedure to accomplish this renders is not complex.
- We start from a tridimensional shape. Then it's discretized in voxels marking as active the ones that are partially or totally included in the volume defined by the surface of the figure.
- Now we are going to discard the non active voxels and we work with the ones that intersect or are included inside the volume.
- We start from a random voxel and we move in a random direction, marking as visited the currant voxel.
- As we move through the voxels we can find a visited voxel in the chosen direction. In this case we chose another direction.
- In the case that there are not any available directions we move backwards and we chose another direction.
- The algorithm ends when all the nodes (voxels) have been visited.
Other views of the cube:





It's possible to use other figures as a "mould" of the labyrinth.
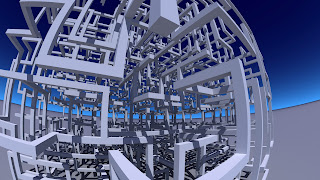
Here we can see a sphere:


And with a spherical camera:

The Utah teapot:

and a some renderings of a lying woman:














